Dark Mirrors: A mathematical look over Arno Schmidt’s fence
Posted: August 22, 2016 Filed under: Literature, People | Tags: Andrew Wiles, Arno Schmidt, Art, Complex numbers, Diophantus, Infinite descent, James Joyce, Leonhard Euler, Literature, Mathematics, Number theory, Philosophy, Pierre de Fermat, Theology, Well ordering principle Leave a commentArt for the people
“Poet: should you receive the applause of the people, ask yourself: what have I done wrong? ! And if your second book is so received as well, then cast away your pen: you can never be great. […] Art for the people? ! : leave that slogan to the Nazis and Communists: it’s just the opposite: the people (everyman!) are obligated to struggle their way to art!“
Arno Schmidt, “Brand’s Heath (1951)

Arno Schmidt (1914 – 1979)
Roaming through the post apocalyptic scenery of Lüneburg Heath in northern Germany, Arno Schmidt’s hero hasn’t met a single soul in five years. The thermonuclear world war that erupted sometime in the mid-fifties between the U.S.A. and the Soviet Union has left the world in ruins and only a few isolated individuals, as the story’s protagonist assumes at some point, are “still nomadizing about”, separated by very large, often contaminated, wastelands. “Dark Mirrors” (1951) is a pessimistic story written mostly in first person narrative, in a diary-like manner, often becoming an inner monologue, bearing the unmistakable mark of Schmidt’s own personality and world views. “The primo motore of it all“, of all evil in the universe, according to Schmidt, is Leviathan, the evil creator, the god-tyrant and predatory monster who created the world in his own image and evilness and for whom the hero of “Dark mirrors” felt “so hate-full, that I raised my rifle, aimed it toward heaven: and through his Leviathan’s maw gaped ten thousand nebulae: I’d like to pounce on the dog!“. In such a world, where Good is unhuman, unnatural and undivine, and contrary to reason, man like “a machine, a mere tool” allows himself to be “used and misused by strange hands […] especially when pressed together in great masses“. Schmidt lets his Crusoe-like alter ego of the “Dark Mirrors” clearly admit it: “if they only had listened to Malthus and Annie Besant; but by 1950 things had gone so far that the earth grew by 100000 every day: one hundred thousand!! I looked contentedly through the black pine stalks: good that it had turned out this way!” The image of the solitary man who gathers his robes about him in defense as he is pressed in by “the bustling, hand-rubbing mob from all directions […] with their hissing, lecherous, paned faces, pointing with mocking, metal-soiled fingers” (“Enthymesis”, 1949), is repeated many times in Schmidt’s writings and in “Dark Mirrors” this man is for once free to roam unperturbed in a much desired solitary utopia where houses, shops, libraries are left almost intact and the mob is removed. “Still small communities left. – The individuals, unaccustomed to the harsh life and raw disease, will quickly die out […] Eventually […] tiny groups may pave the way for a repopulated earth; but that will take – well – let’s hope a thousand years. And that’s all to the good!” For Schmidt’s hero that was a much despised humanity, where the mob had no vision and no higher ideals: “Boxing, soccer, the lottery: how those legs did run! – Very big when it came to weapons! – What were a boy’s ideals: auto-racer, general, world-champion sprinter. A girl’s: film star, ‘creator’ of fashion. The men’s: harem owner and manager. The woman’s: car, electric kitchen, to be addressed as ‘milady’. The old codgers’: statesman –“. Reason, Science, Culture, Morality, all these were left to a tiny enlightened elite who lived in parallel: “Culture!?: one in a thousand passed culture on; one in a hundred thousand created culture!: Morality?: Hahaha!: Let every man prove his conscience and say he wasn’t ripe for hanging long ago!” Therefore the artist “should thumb his nose at the taste and niveau of the public“.
Artists and Scientists
It is not a surprise that reading Schmidt’s works is a kind of struggle as, apart from the avant-garde, experimental means of narration and even his peculiar use of language and spelling (rendering much of Schmidt’s work, just like Joyce’s, virtually untranslatable), the author seems to be asking from his readers to do their homework first, in History, in Literature, in Philosophy, in Theology and, quite often, in Mathematics, in order to approach his texts. In Schmidt’s “religion”, morality and pure reason, as expressed by Mathematics, coincide: “[…] no form of stupidity, vice, or wickedness can be invented, whose illogicality or perniciousness has not long ago been proved just as rigorously as any theorem of Euclid: and nevertheless! Notwithstanding, human beings have kept on spinning about in the very same circle of stupidity, error, and abuse for several thousand years, growing no wiser either through their own experience or that of others […]” (“Dark Mirrors”). Though Schmidt had not received any formal mathematical education, he displayed keen amateur interest in Mathematics and for some years, while employed in a textile factory as a stock accountant, he even worked privately in compiling tables of logarithms with ten correct digits, a rather futile, tedious and unrewarding occupation. Schmidt’s writings are abundant with allusions and direct or indirect references to Mathematics with concepts, theorems, even formulae, explicit puzzles, problems and proofs: these sometimes impenetrable mind games, certainly not at the taste of the vast public, serve as another technique in building his artistic ramparts. But beyond that, clearly reflecting his personal philosophy, one can sense in his writings an atmosphere of admiration and reverence towards the Arts, Mathematics and Science in general, contrary to the contempt displayed towards the great leaders, warriors and conquerors: “Who can only be great?” notes Philostratus in “Enthymesis” (1949). “Artists and Scientists! Nobody else! And amongst them, the humblest is a thousand times greater than the great Xerxes”.
Man in the universe
In “Dark Mirrors”, after a description of the wanderings of the story’s hero in silent ghost towns, where life has apparently stopped abruptly, leaving behind mostly intact houses with their inhabitants’ skeletons sometimes still in them, he wakes up in the middle of the night and his mind is fascinated by a quite extraordinary metaphor, a “pretty and clever little mind game; for 5 minutes”:
“Reciprocal radii (and the notion fascinated me for 5 minutes). – Imagine the graphic representation of functions with complex variables, and in particular, the special case just mentioned: a most apt symbol of man in the universe (for he is the unit-scale circle in which All is mirrored and whirls and is reduced! Infinity becomes the deepest, internal centerpoint, and through it we cross our coordinates, our referential system and measure of things. Only the peripheral skin is equal to itself; the borderline between macro and micro.-“
Remarkably and unpoetically, human condition with respect to the universe is likened here to an abstract concept from the mathematical world, from the pure world of scientific thought (and one that is out of immediate reach for most readers), despite the reality the hero is facing and despite the fact that this reality is the result of the advances of this exact scientific thought gone astray. Yet Schmidt has quite clear in his mind who’s to blame and for him Science, Mathematics and reason are simply abused and misused “by the strange hands” of Leviathan himself, or the hands of humans contaminated by Leviathan’s genome:
“If only humankind would soon succeed in destroying itself: true, I’m afraid: it will take a long time yet, but they’ll manage it for sure. […] For they pervert all things to evil. The alphabet: it was intended to record timeless poetry or wisdom or memories – but they scrawl myriads of trashy novels and inflammatory pamphlets. What do they deftly make of metals? Swords and arrow tips. – Fire? Cities are already smoldering. And in the agora throng the pickpockets and swashbucklers, cutpurses, bawds, quacks and whores. And at best, the rest are simpletons, dandies, and brainless yowlers. And every one of them self-complacent, pretending respectability, bows politely, puffs out coarse cheeks, waves his hands, ogles, jabbers, crows.” (“Leviathan – Enthymesis”, 1949)
Still, Schmidt’s “reciprocal radii” metaphor might remain to most an impenetrable puzzle, a question mark, one of those mind games and obstacles so often found in Schmidt’s writings, one that compels most of his readers to simply recognize it as another eccentricity which doesn’t necessarily have to be resolved, especially as it exceeds by far the literary sphere, and simply proceed. Yet, for him “the people (everyman!) are obligated to struggle their way to art” and in his writings he continually puts his readers to the test, applying his gasket of “grids”, “photo albums” or “mind games” (as he called his techniques) to separate those who are willing to struggle their way from those who don’t.
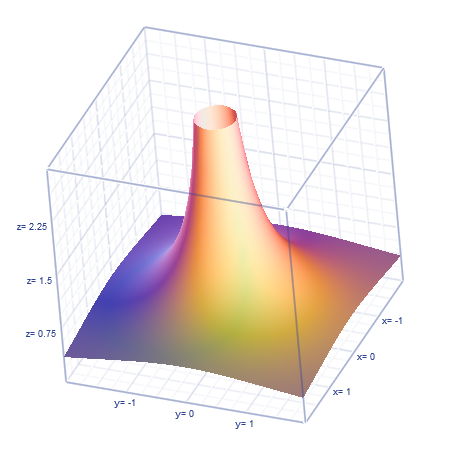
The function f(w)=1/|w| (Arno Schmidt’s metaphor for man in the universe)
Infinite descent
Further along “Dark Mirrors” the test becomes even more demanding: in the last paragraph of part I Schmidt makes an ironic mention of the notorious “last theorem of Fermat” (named thus as this was the last unresolved issue out of Fermat’s work), a problem that remained unsolved for more than three centuries and was still unsolved at the time Schmidt’s story was written. While narration time has accelerated, squeezing whole months within a couple of lines, the text suddenly stops and focuses on the hero’s enthusiastic attempt of solving the problem. The irony is aimed against the nature of humanity that, under the commands of the predatory creator, has deployed the technological and scientific advances to destroy itself, leaving behind unresolved even the fundamental, emblematic problems of thought. And this might be one of the last attempts, left on an amateur and layman. What follows, though informal, is actually a mathematical text:
“November second broke off the leaves, sheets of copper lay all about, one fiery week long; then I vanished into the early and hard winter (another match with the eleven-year sunspot cycle, wasn’t it?!) In January the brook froze and I had to melt a lot of ice; the stove thundered and gave a broad-hipped glow by white-blue day and by zebraed night.
The moon flashed sharp shadows about me and appeared ever and again in its velvet abysses. Once a stormwind blew from the east for 50 hours straight, and the reading was twenty-eight below. (That same morning an occultation of Jupiter occurred.)
The black dome of night: from the orbicular upper light at the zenith it came, toxic clear and so jeering bright that the snow burnt eyes and soles. I sat down on my two top wooden steps, and wrote on a large sheet:
Fermat’s theorem: If AN+BN=CN, given whole natural numbers, N can never be larger than 2. I quickly proved this for myself as follows:
(1) AN=CN-BN or A2·N/2=(CN/2-BN/2)·( CN/2+BN/2) , therefore
(2) AN/2=the root of the right side; let CN/2-BN/2=x2 and CN/2+BN/2=y2, that automatically give
(3) AN=(x·y)2=a2 and further it is demonstrated that:
(4) CN=[(x2+y2)/2]2=c2 just as
(5) BN=[(y2-x2)/2]2=b2
The equation AN+BN=CN can therefore always be reduced to the quadratic equation a2+b2=c2, wherein x and y are fundamental integers. For a, b and c to be whole numbers, x and y must likewise be whole numbers, besides which y-x=2m etc. etc. (And immediately several possibilities: for y=4; x=2 the result is 82+62=102. For y=5; x=3, the result is 152+82=172; so that an 8 can appear twice, depending on whether it is a or b).
And now for the Meaningful General: in the case of whole numbers, every expression of AN+BN+CN+DN+……=ZN may in its simplest form have N elements on the left side, no less! And – as above with the example of 8 – the same number value may occur at most N times, depending on whether it is AN, BN, etc. E.g. for N=3 the result is: 33+43+53=63; 183+33+243=273; 363+373+33=463. The symbols drew themselves out nimbly from my pencil, and I bungled merrily along: just imagine that: I’m solving the problem of Fermat’s theorem! (But time flew in exemplary fashion all the while).“
The casual manner by which a “quick proof” is launched is probably an allusion to Fermat’s own note in the margin of a copy of Diophantus’ “Arithmetica” (3rd century A.D.): “It is impossible to separate a cube into two cubes, or a fourth power into two fourth powers, or in general, any power higher than the second, into two like powers. I have discovered a truly marvelous proof of this, which this margin is too narrow to contain“. These couple of lines haunted the mathematical community for three and a half centuries, remaining one of the biggest mathematical mysteries (or the biggest and most ingenious prank ever played upon mathematicians by one of the great masters) as this “marvelous proof“, and actually any proof, marvelous or not, remained elusive. It was only in 1994 that Fermat’s last theorem became eventually a formal theorem by the mathematician Andrew Wiles (1953-) who, after tedious efforts, composed a proof within a few hundred pages, making use of advanced mathematical concepts unavailable to Fermat. Even to present day, and even more in Schmidt’s time when the problem was still unresolved, some struggle to concoct a marvelous little proof like the one promised by Fermat in his margin note, one that could be laid out neatly with Mathematics available in Fermat’s time, maybe in just a couple of sheets, launching simplistic efforts not very different in nature than that of the hero of “Dark Mirrors” who, sitting on his “two top wooden steps“, he “bungles merrily along“. What Schmidt presents here is strongly reminiscent of Fermat’s ingenious “infinite descent” approach for proving that there is no right triangle with square area (given that the sides of the triangle have integer lengths), which indeed is equivalent to proving the special case of Fermat’s last theorem for N=4. “Infinite descent” employs the “well ordering principle” (the simple fact that there is a least natural number and therefore one cannot continue climbing down the ladder of whole numbers without reaching an end) to arrive at a contradiction: if a right triangle has a square area then it is proved that necessarily exists another, smaller right triangle with square area and after that still another etc. ad infinitum. But this is a contradiction as, given that all these triangles have sides with integer lengths, this climbing down cannot continue for ever. It seems that Schmidt was quite aware of this technique and possibly intrigued by how well the name of the method, “infinite descent”, expresses key concepts in “Dark Mirrors”.
The “Meaningful General” that follows is only the obvious generalization: if Fermat’s last theorem holds for sums of two powers, then why not similarly for sums of three or more powers etc.? Therefore, within whole (natural) numbers, it seems that N in AN+BN+CN=DN should never be larger than 3, N in AN+BN+CN+DN=EN should never be larger than 4 etc. This is known as Leonhard Euler’s (1707 – 1783) conjecture and was disproved in 1966 by Leon J. Lander and Thomas Parkin who, using a computer, provided a single counterexample:
275+845+1105+1335=1445
Schmidt has thus his hero bungling merrily along two of the most famous still unsolved problems of Mathematics at the time the “Dark Mirrors” was written, describing a world where humanity is on the brink of extinction yet even fundamental, emblematic problems of thought remain dishonorably unresolved.
The highest fence
In 1958 Schmidt and his wife moved away from the city environment of Darmstadt to a small house in Bargfeld, Lower Saxony, in the countryside, which was destined to be his home until his death on June 3, 1979. He settled his desk and his books in the attic, constructed a garden door of his own design and erected a fence made of planks and wire around his property, just as he did in his writings, using experimental prose forms, peculiar language and spelling and even mind games and mathematical concepts. His home had no doorbell. He dedicated all of his time to his work, producing “Zettel’s Traum” (written over a period from 1963 to 1970), his magnum opus, a huge 1334-page, virtually unreadable, virtually untranslatable, seemingly disorganized text that was by some described as an imitation of “Ulysses” and “Finnegans Wake” but also as “the literary masterpiece of the century; but it could be also a real size matchstick Eiffel tower created by an amateur out of his mind at the great cost of his own life – maybe it’s both” (Dieter E. Zimmer, “Time” magazine, May 1970). It seems that this was the highest fence he ever erected around him. Today he is considered one of the greatest writers in German language of the post-war era.